	Algebra Tutorials!

	Thursday 21st of November



	Home
	Scientific Notation
	Notation and Symbols
	Linear Equations and Inequalities in One Variable
	Graphing Equations in Three Variables
	What the Standard Form of a Quadratic can tell you about the graph
	Simplifying Radical Expressions Containing One Term
	Adding and Subtracting Fractions
	Multiplying Radical Expressions
	Adding and Subtracting Fractions
	Multiplying and Dividing With Square Roots
	Graphing Linear Inequalities
	Absolute Value Function
	Real Numbers and the Real Line
	Monomial Factors
	Raising an Exponential Expression to a Power
	Rational Exponents
	Multiplying Two Fractions Whose Numerators Are Both 1
	Multiplying Rational Expressions
	Building Up the Denominator
	Adding and Subtracting Decimals
	Solving Quadratic Equations
	Scientific Notation
	Like Radical Terms
	Graphing Parabolas
	Subtracting Reverses
	Solving Linear Equations
	Dividing Rational Expressions
	Complex Numbers
	Solving Linear Inequalities
	Working with Fractions
	Graphing Linear Equations
	Simplifying Expressions That Contain Negative Exponents
	Rationalizing the Denominator
	Decimals
	Estimating Sums and Differences of Mixed Numbers
	Algebraic Fractions
	Simplifying Rational Expressions
	Linear Equations
	Dividing Complex Numbers
	Simplifying Square Roots That Contain Variables
	Simplifying Radicals Involving Variables
	Compound Inequalities
	Factoring Special Quadratic Polynomials
	Simplifying Complex Fractions
	Rules for Exponents
	Finding Logarithms
	Multiplying Polynomials
	Using Coordinates to Find Slope
	Variables and Expressions
	Dividing Radicals
	Using Proportions and Cross
	Solving Equations with Radicals and Exponents
	Natural Logs
	The Addition Method
	Equations

Try the Free Math Solver or Scroll down to Tutorials!

Enter expression, e.g. (x^2-y^2)/(x-y)

Enter expression, e.g. x^2+5x+6

Enter expression, e.g. (x+1)^3

Enter a set of expressions, e.g. ab^2,a^2b

Enter equation to solve, e.g. 2x+3=4

Enter equation to graph, e.g. y=3x^2-1

Depdendent Variable

Number of equations to solve:

Equ. #1:

Equ. #2:

Equ. #3:

Equ. #4:

Equ. #5:

Equ. #6:

Equ. #7:

Equ. #8:

Equ. #9:

Solve for:

Enter inequality to solve, e.g. 2x+3>4

Enter inequality to graph, e.g. y<3x^2-1

Dependent Variable

Number of inequalities to solve:

Ineq. #1:

Ineq. #2:

Ineq. #3:

Ineq. #4:

Ineq. #5:

Ineq. #6:

Ineq. #7:

Ineq. #8:

Ineq. #9:

Solve for:

Math solver on your site

Please use this form if you would like
to have this math solver on your website,
free of charge.

Name:

Email:

Your Website:

Msg:

Absolute Value Function

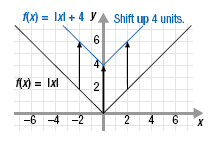
To graph an absolute value function such as f(x) = |x| + 4 we could calculate several ordered pairs, plot them, and then connect the points. However, the graph of f(x) = |x| + 4 is related to the graph of f(x) = |x|. It has the same characteristic shape, but the +4 outside the absolute value symbols shifts the graph up 4 units.

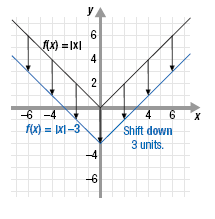
Likewise, the graph of f(x) = |x| + 3 is the same as the graph of f(x) = |x| but shifted down 3 units.

Example

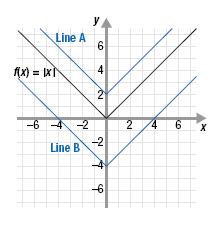
Determine the equation of Line A and Line B shown in the graph.

State the domain and range of each function.

Solution

Line A is the graph of f(x) = |x| shifted up 2 units. Therefore, its equation is f(x) = |x| + 2. The domain is all real numbers; the range is y ≥ 2.

Line B is the graph of f(x) = |x| shifted down 4 units. Therefore, its equation is f(x) = |x| - 4. The domain is all real numbers; the range is y ≥ -4.

When a constant is added or subtracted inside the absolute value symbols the graph of f(x) = |x| is also shifted, but this time to the left or right.

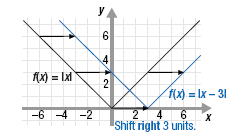
For example, the graph of f(x) = |x - 3| has the same shape as that of f(x) = |x| but it is shifted 3 units to the right.

Likewise, the graph of f(x) = |x + 4| has the same shape as f(x) = |x| but it is shifted 4 units to the left.

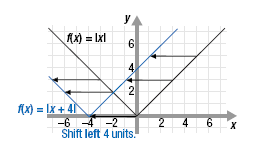
Be careful.

The graph of y = |x - 3| is shifted 3 units to the right; that is, in the positive direction along the x-axis. This may be the opposite of what you expect.